
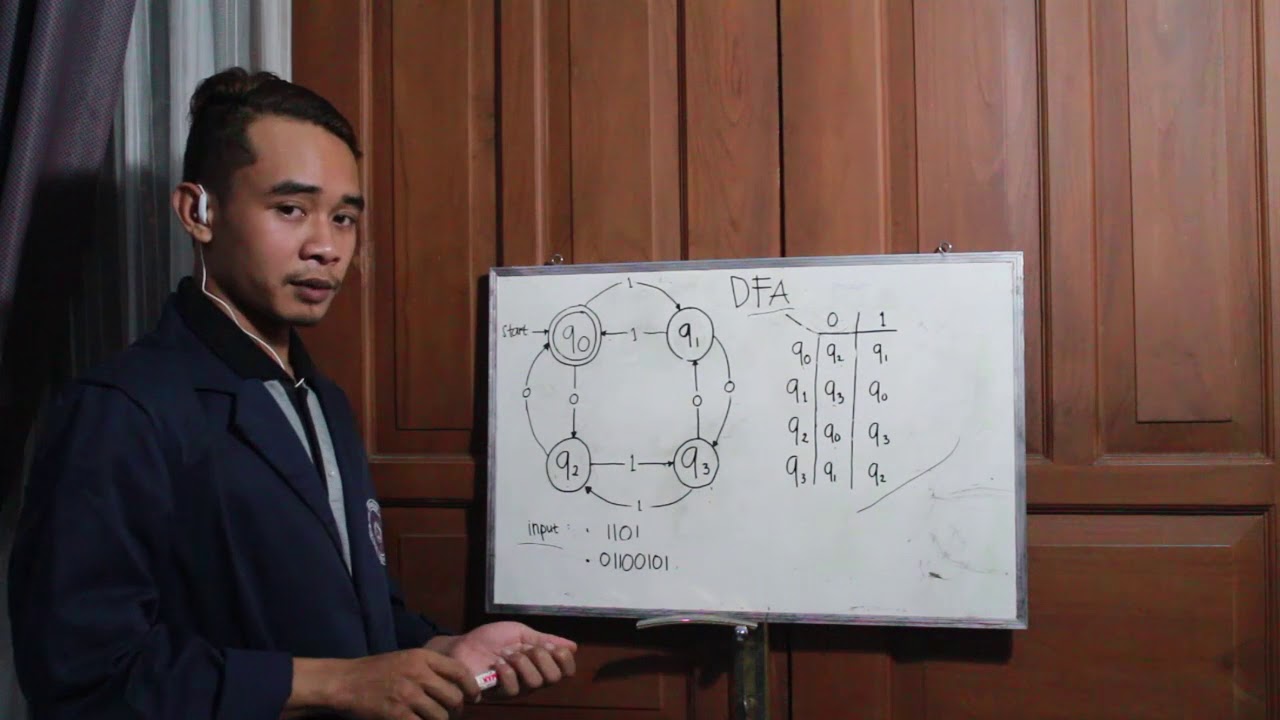
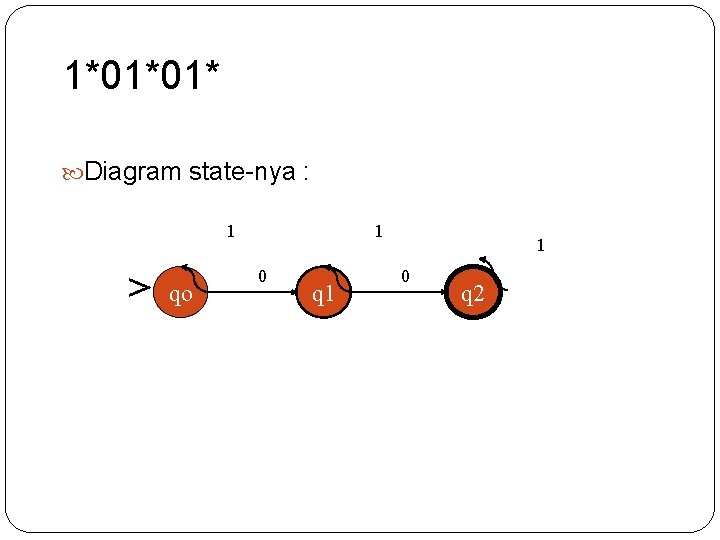
Busch - LSUĮkuivalensi NFA-ε Dengan ER (Ekspresi Regular) NFA- ε = NFA-λ (Automata Hingga Non-deterministik = NFA) atau λ atau r1 ˅ r2 atau r1 + r2 Prof. When we say: We are given a Regular Language We mean: Language is in a standard representation (DFA, NFA, or Regular Expression) Prof. materi kuliah teori bahasa dan otomata oleh. Standard Representations of Regular Languages Regular Languages DFAs Regular Expressions NFAs Prof. ekspresi regular ekspresi regular materi minggu materi minggu ke ke-4 4. Busch - LSUīy repeating the process until two states are left, the resulting graph is Initial graph Resulting graph The resulting regular expression: End of Proof-Part 2 Prof. Reducing the states: Transition labels are regular expressions Prof.

Busch - LSUĪnother Example: Transition labels are regular expressions Prof. Busch - LSUįrom construct the equivalent Generalized Transition Graph in which transition labels are regular expressions Example: Corresponding Generalized transition graph Prof. Since is regular, there is a NFA that accepts it Take it with a single accept state Prof. Proof - Part 2 Languages Generated by Regular Expressions Regular Languages For any regular language there is a regular expression with We will convert an NFA that accepts to a regular expression Prof. Using the regular closure of these operations, we can construct recursively the NFA that accepts Example: Prof. Therefore: Are regular languages is trivially a regular language (by induction hypothesis) End of Proof-Part 1 Prof. We also know: Regular languages are closed under: Union Concatenation Star By inductive hypothesis we know: and are regular languages Prof. Busch - LSUīy definition of regular expressions: Prof. Inductive Step We will prove: Are regular Languages Prof. Inductive Hypothesis Suppose that for regular expressions and, and are regular languages Prof. Regular languages Induction Basis Primitive Regular Expressions: Corresponding NFAs Prof. Busch - LSUįor any regular expression the language is regular Proof by induction on the size of Proof - Part 1 Languages Generated by Regular Expressions Regular Languages Prof. Proof: Languages Generated by Regular Expressions Regular Languages Languages Generated by Regular Expressions Regular Languages Prof. Theorem Languages Generated by Regular Expressions Regular Languages Prof. View 05- EKSPRESI REGULAR- TB&O -STT PB August2019 Upload1.ppt from EEN 15 at President University. Regular ExpressionsandRegular Languages Prof. Busch - LSUĮxample Regular expression Prof. Busch - LSUĮxample Regular expression: Prof. Busch - LSUĭefinition (continued) For regular expressions and Prof. Busch - LSUĭefinition For primitive regular expressions: Prof. Languages of Regular Expressions : language of regular expression Example Prof. Not a regular expression: Examples A regular expression: Prof. Given regular expressions and Are regular expressions Recursive Definition Primitive regular expressions: Prof. Regular Expressions Regular expressions describe regular languages Example: describes the language Prof.


 0 kommentar(er)
0 kommentar(er)
